Socolar and Taylor’s aperiodic tile.
As regular readers know I have a love of incomplete or impossible quests. One such quest that comes very close to my work is quest for an aperiodic tile. A shape that can tile the plane, but not periodically. In other words I can cover whatever area I like (the floor of the room or the Milky Way Galaxy) but the tiling will never be a single patch moved about by translations. A big development, a new tile from Joshua Socolar and Joan Taylor, is therefore very exciting, especially as the final answer is still illusive.
For a long time it was thought that even for collections of tiles could not force non-periodicity. Then in the 1960s work in logic revealed that they could and the study of aperiodic tilings began. Today the phenomena is still not very well understood. There are only a few known aperiodic sets of shapes with a small number of tiles (though if you let the number of tiles get large an infinite number of examples are known). Even for the known examples, such as the famous Penrose tiling, the proof that the tiles cannot tile in any periodic manner involves simply putting shapes together and showing patches that can and cannot occur. The Penrose tiling obviously uses two tiles, which leaves the quest: can it be done with one? So we come to the new tile:
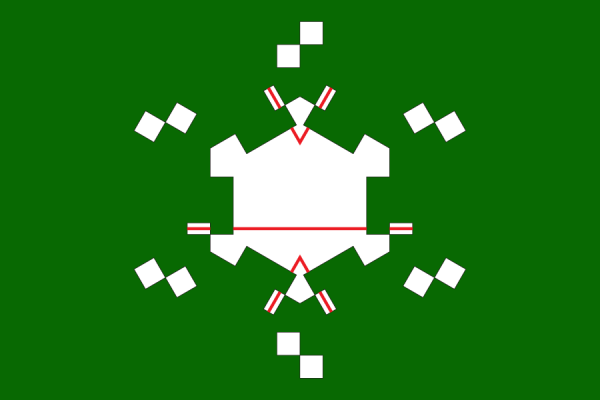
This tile can tile the plane, but not in any periodic manner. This is enforced just by the shape of the tile. The red lines above are not necessary, but reveal a little of the structure of the tilings:
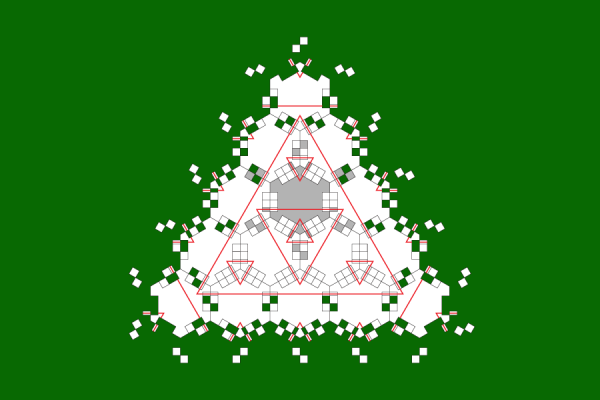
Patch of tiling, the red lines show a little of the structure of the tiling. The single grey tile shows how the different pieces of the tile fit together with its neighbours.
As you can see from the patch above the tile forces the formation of a sort of Sierpinsky triangle. It is this structure, occuring at all scales, that ensures that the tilings produced cannot be periodic.
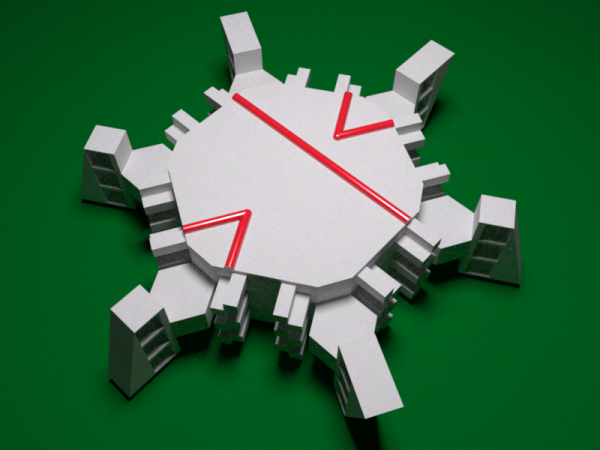
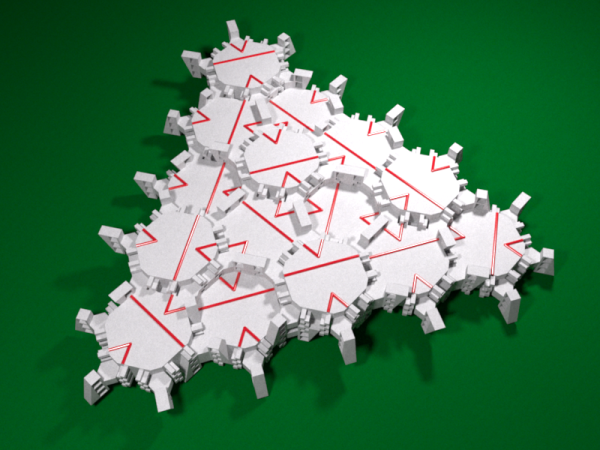
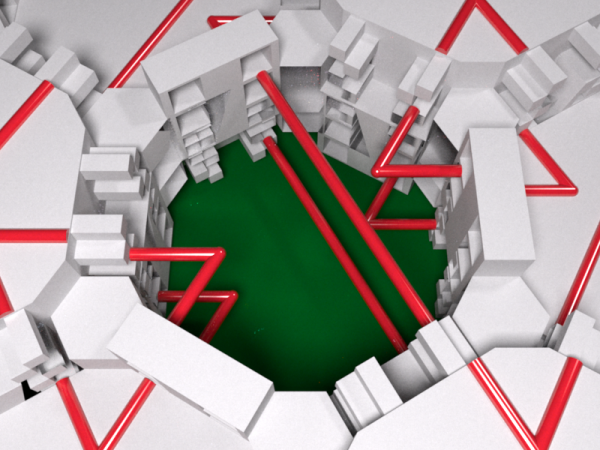
You might have noticed that the tile shown above is slightly unusual. It is made up of several disconnected parts. This leaves a question open, is there a connected aperiodic tile? I would like this as the current tile is hard to make on the laser cutter! A possible way to solve this problem is to use a third dimension, and this is what Socolar and Taylor do, giving the tile shown at the start of this post. We can take copies of this tile and build patches, again the hierarchical triangles appear (note that the tiles fit together at different levels):
The larger space allow information on the tile edges to cross, as you can see in this patch with one tile removed:
This tile therefore, is connected and can lie in no periodic tilings of 3d. However this is not quite the whole story, although the tilings produced will never use a finite region translated around they will only be non-periodic in two directions. In the third direction the tiling can be periodic.
For more details look at Socolar and Taylor’s paper and Joan Taylor’s original paper (complete with beautiful hand-drawn images). The story has also been covered by the MAKE blog and Technology Review. If you want to make your own images and even tiles I have put my tile models up on Thingiverse and also put the blender file online.


Pingback: Socolar-Taylor aperiodic tile models on Thingiverse | Products & Tech News
Pingback: Socolar-Taylor aperiodic tile models on Thingiverse « The tech telecom and all
That was a really good blog post,I recently subscribed to your feed.
Pingback: I find myself looking for a job… « Maxwell's Demon
Pingback: Tile Sales
Pingback: The top 10 mathematical achievements of the last 5ish years, maybe | Simple City
Pingback: Snowflake, Seashell, Star | Maxwell's Demon
Pingback: The top 10 mathematical achievements of the last 5ish years, maybe – Mathematics in Europe